Insights into Instabilities
Holmes’ research looks at how soft materials buckle, snap, and jam
By Liz Sheeley
Even with the deep scientific understanding surrounding of how matter behaves in many different scenarios, scientists are still learning about how new or more unusual types of structures, materials, and systems, that are becoming more prevalent, react to little understood forces. Soft matter, which is anything from gels to liquids to polymers, falls into this group of matter that behaves in nuanced ways that are still not yet fully defined. In two recent papers published in Physical Review Letters, Assistant Professor Douglas Holmes (ME, MSE) outlines two different concepts that push forward our understanding of how soft matter behaves under unstable conditions like growth.
Holmes spends his time studying how thin structures behave—their physics, geometry and mechanics. With the invention of materials that are super thin, but also super strong, engineers have started to use them to build objects like car accelerometers or lab-on-a-chip within the world of mircroelectromechanical systems (MEMS). But problems arise when a non-mechanical force called natural curvature buckles a thin shell significantly earlier than engineers would predict based on a century-old pressure-buckling model.
Natural curvature occurs when an object grows or swells. There is a species of bacteria that grows inside out—so when it matures, it has to completely invert. The bacteria does this by natural curvature. And if a thin curved shell experiences natural curvature, it buckles earlier than scientists would predict. This is because although pressure and natural curvature behave in a similar way to create instabilities, they are not the same.
“The problem with all thin structures — shells, plates, beams — is that they’re really, really strong until they fail and they can fail by buckling, and they can fail in a catastrophic way,” says Holmes. “A long-standing problem in mechanics has been trying to predict when a spherical shell will buckle. There’s a very classical predication for shells under pressure that was made over 100 years ago. But we show that the point at which a shell buckles under natural curvature, although similar to pressure buckling, is actually very different.”
In one of his recent papers, Holmes and his team, along with a collaborator from the Massachusetts Institute of Technology, studied how a thin shell responded to swelling, which is how the researchers mimicked growth in the lab. Holmes uses two different polymers on either side of the shell and they tune the amount of natural curvature using a technique they developed called residual swelling.
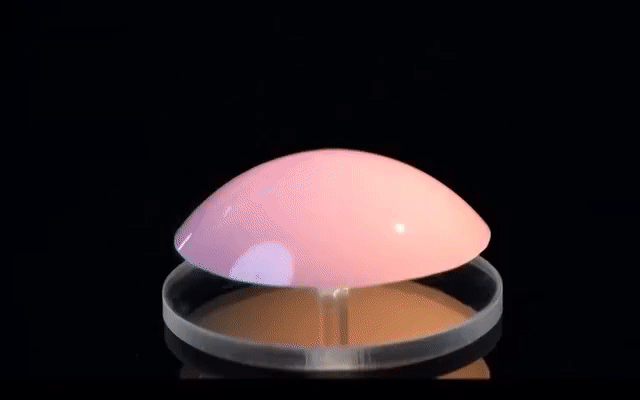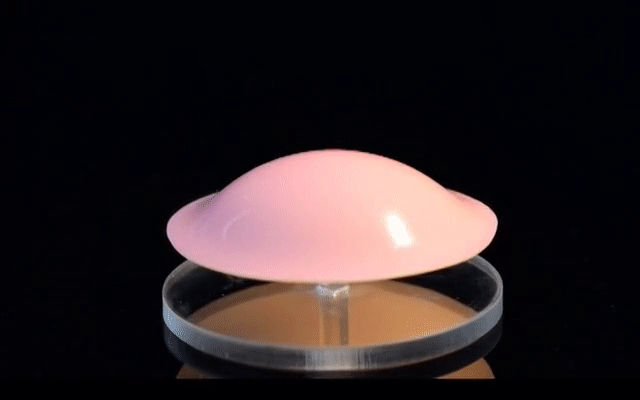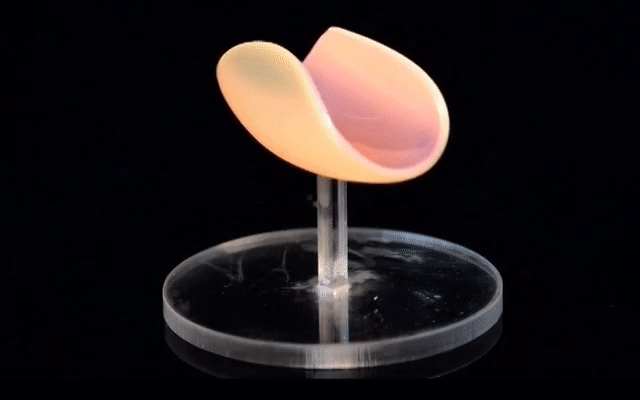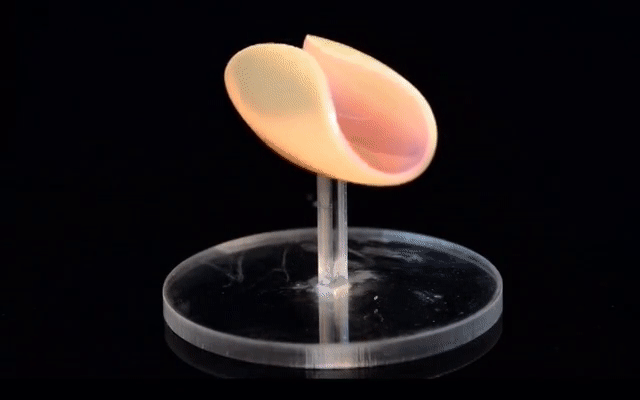
“The pink polymer and the green polymer are very, very similar,” says Holmes. “The only difference between the two of them is that the pink polymer has extra fluid in it; you can kind of think of it like a wet sponge. The green polymer is kind of like a dry sponge and when you stick them together some of the fluid goes from the wet sponge to the dry sponge until they’re both equally wet. That means the pink one gets smaller because it just lost some material and the green one gets bigger.”
As the shell swells, it curves up or down depending on if the inside or outside of the shell is swelling. If the outside is swelling (the green polymer is on the outside), then the shell will curve down; if the inside is swelling, it will curve up. This spontaneous curvature intrigues Holmes.
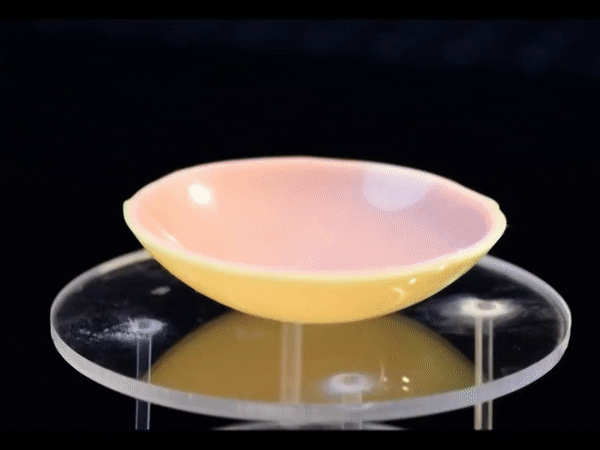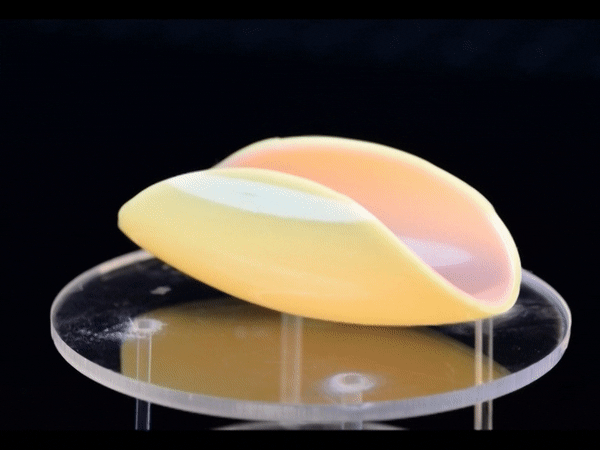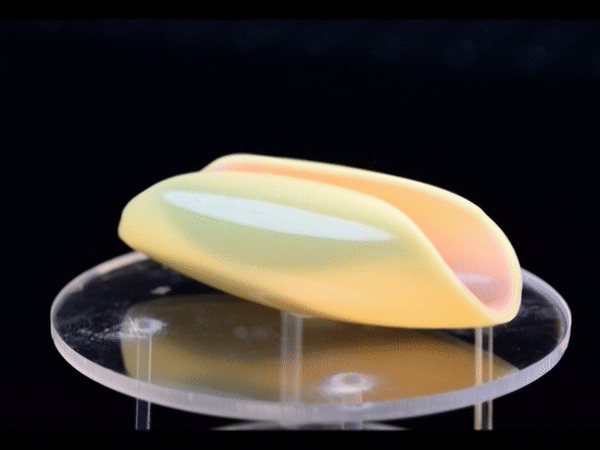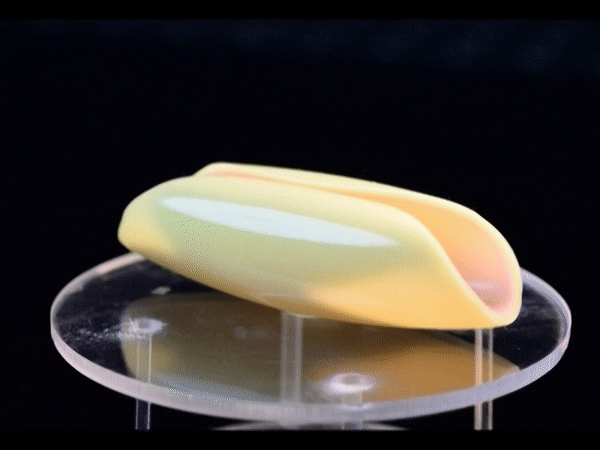
“An instability called buckling is one of the first concepts that mechanical engineers learn about; buckling is one way that objects fail when they’re thin,” says Holmes. “A different instability is called snapping. Snapping is like when your umbrella gets turned inside out on a windy day; buckling is like when you crush a soda can.”
The surprising thing Holmes and his colleagues discovered is the difference between how and when a shell becomes unstable depends on whether the swelling creates an upward or downward curvature. Holmes said that this paper shows that if an object is buckling, the geometry of that object doesn’t help predict the point at which it will buckle. But if an object is snapping, the object’s geometry matters a lot. This allows scientists to predict when a curved object will buckle or snap depending on the type of natural curvature it is subjected to.
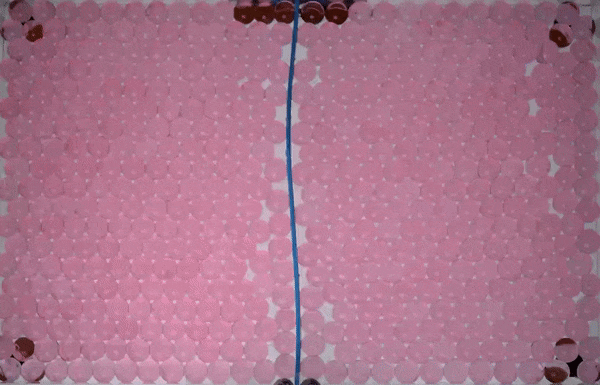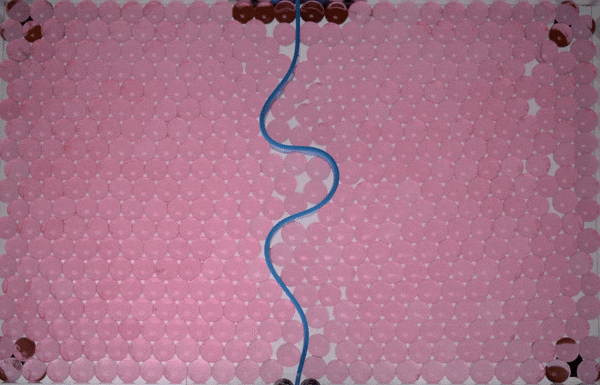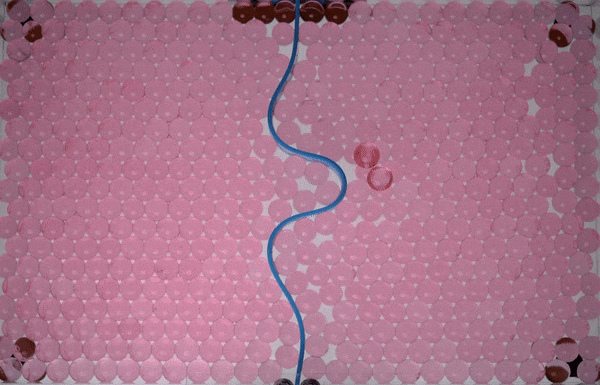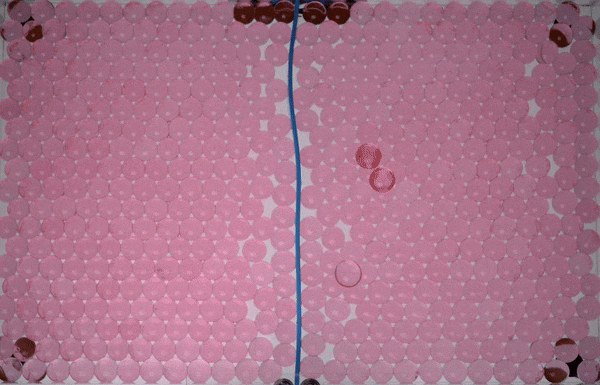
The second piece of work, published as the cover story in Physical Review Letters in February 2018, explores a different concept. Holmes and his lab investigated the mechanics behind what happens when a slender rod is pushed into granular materials. To study this, they built a box, put differing amounts of small beads into it, and observed what happened when they inserted a rod.
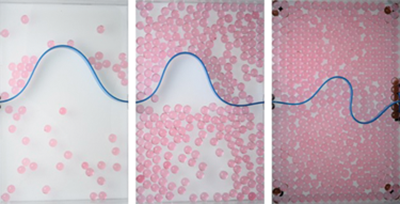
To think about this concept outside of the lab, the rod can represent a tree root and the granular material can be soil. When a root is growing, it will navigate itself through the soil, but will change direction if it encounters resistance. What Holmes studied is what point does the root “win out” and grow through the soil, and on the opposite side, what point does the soil “win out” and the root redirects itself.
The experiment detailed when the grains acted as a gas, liquid or solid, depending on how many grains there were in the box. The results were twofold: the team can determine how far a beam can be pushed into a specific density of a granular material before it begins to feel the pressure of the grains pushing back onto it; they also showed that the beam and the grains each had an effect on each other.
Holmes says that in mechanics, there is a concept called a diverging length scale which can measure how jammed a system is at a point in time. For the beam, this means measuring how close together the two bumps are that are formed when the beam is bending to the will of the grains; and for the grains it means measuring how much they can move. Without any grains in the box to push back on the beam, it would make one curve so the object is curved the same amount throughout its length. But when the grains create an obstacle, the beam curves and forms two smaller bumps.
“To measure how confined the beam is, you measure the length in the beam between the two bumps,” says Holmes. “Then we can calculate the rate at which the beam is jamming, or its diverging length scale. If we just measured roughly how many grains are moving as we inserted the beam, the number of grains that are moving drops at the same rate that the beam jams, and we don’t know why.”
Even in the simplest case of an elastogranular system of a beam and beads in a box, everything is coupled. What the beam does is completely dependent on the grains, whether that is their orientation, their ordering, how jammed or not jammed they are; and everything that the grains are doing is dictated by how stiff the elastic beam is, and where it’s able to bend and move.
This concept also has an application in smart needles. These findings detail that what a needle could do or how it could move, is dependent on the tissue it’s being inserted into. “The object can sense its surroundings really well,” says Holmes. “In theory you could use that to let the object sense resistance and generate feedback to tell the object to bend away from an area that is stiffer.”
“The second paper is almost opposite to the first one,” says Holmes. “The first one presented a very complete story of a problem, but the second one opens many more questions than we’ve answered.” But together, the papers offer new perspectives and understandings on the mechanics of thin structures to expand scientific knowledge and move the conversation forward.