R. Babich, J. Howard, C. Rebbi, N. Shoresh, Department of Physics and Center for Computational Science, Boston University, USA
C. Hoelbling, Department of Physics, Bergische Universitat Wuppertal, Germany
N. Garron, L. Lellouch, S. Necco, Centre de Physique Theorique, Marseille, France
F. Berruto, Department of Physics, Brookhaven National Laboratory, USA

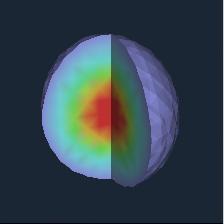
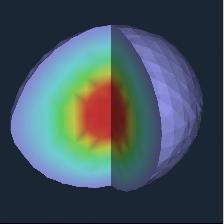
Quantum Chromodynamics (QCD), the theory of nuclear constituents, is simulated using the “overlap” formalism for putting quarks on a space-time lattice.
The overlap formalism preserves chiral symmetry, a crucial symmetry of continuum QCD, but at a heavy computational cost: to find the quark propagator one must evaluate D(D † D)-1/2χ, where χ is a complex vector with over 5 million components and D is a sparse matrix in the same space (i.e. 5 million x 5 million complex).
The overlap formalism preserves chiral symmetry, a crucial symmetry of continuum QCD, but at a heavy computational cost: to find the quark propagator one must evaluate D(D†D)-1/2?, where ? is a complex vector with over 5 million components and D is a sparse matrix in the same space (i.e. 5 million x 5 million complex).
Krylov space methods and other advanced computational techniques are used to solve the problem and thus calculate masses and other properties of particles made of quarks.
